 Sideway
BICK BLOG from Sideway
Sideway
BICK BLOG from Sideway
|
Link:http://output.to/sideway/default.asp?qno=130600005 Pythagorean identities , Pythagorean Conjugates, Angles Sum and Difference Identities, Common Identities, Properties of Trigonometric Functions TrigonometryThose fundamental trigonometric identities are related with the properties of trigonometric functions. Some trigonometric identities or Laws are derived as mathematical equalities that are always true for used with trigonometric functions simlificaton and transformation . The Pythagorean identities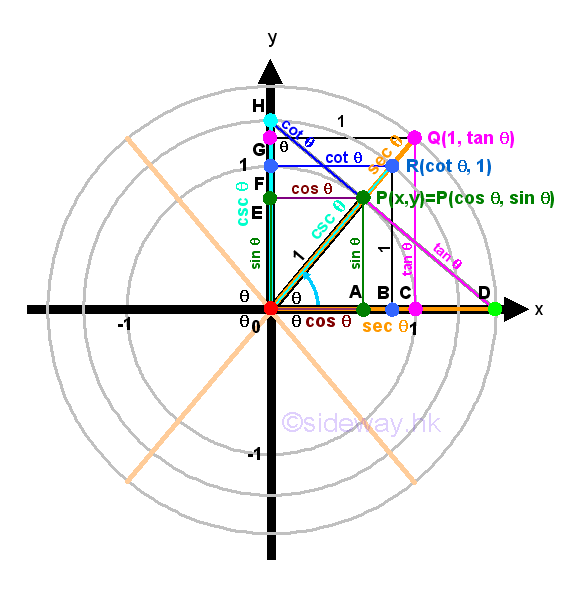
As the point P, lied on the unit circle, can be viewed as the leg of a right-angled triangle ΔPOA with hypotenuse equal to 1, the Pythagorean theroem can be applied, that is x2+y2=z2. Substituting x=cos(θ), y=sin(θ) and z=1 into the equation x2+y2=1. Imply (cos(θ))2+(sin(θ))2=1 and for convenience the identity is written as cos2(θ)+sin2(θ)=1. The identity implies the theorem is always true for any angle θ. Impy 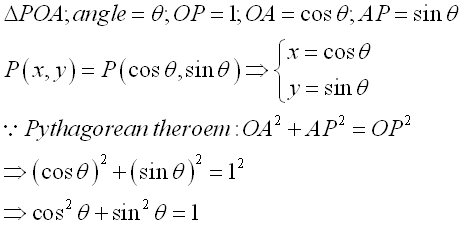
The identity can be used to determine the unknown term up to a sign, ±, when either the cos(θ) or sin(θ) is known. The ambiguity of the sign, ±, can be removed if the terminal side of angle θ lies on the coordinate system in standard position is known. Impy 
Besides the most important pythagorean identity of sine and cosine functions, other pythagorean identities can also be obtained by Pythagorean theorem from other similar right-angled triangles ΔQOC and ΔROB, that is 1+tan2(θ)=sec2(θ) and cot2(θ)+1=csc2(θ) . Imply 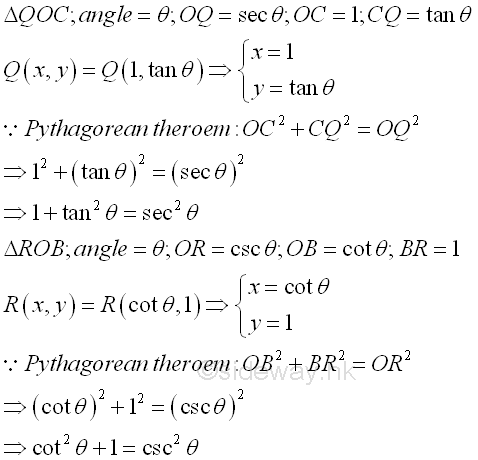
Or derived from the first pythagorean identity. Impy 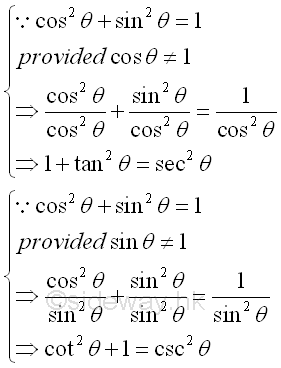
The Pythagorean ConjugatesSince Pythagorean identities are made up of squares, Pythagorean identities can also be transformed to other forms called the Pythagorean Conjugates by making use of Difference Of Squares. Through rearrangement of the Pythagorean identity to the form of difference of squares, a Pythagorean identity can then be factored into two factors accordingly. That is cos2(θ)+sin2(θ)=1 becomes 1-cos2(θ)=sin2(θ) or 1-sin2(θ)=cos2(θ), imply (1+cos θ)(1+cos θ)=sin2(θ) or (1+sin θ)(1+sin θ)=cos2(θ). And 1+tan2(θ)=sec2(θ) becomes sec2(θ)-tan2(θ)=1, imply (sec θ+tan θ)(sec θ-tan θ)=1. And cot2(θ)+1=csc2(θ) becomes csc2(θ)-cot2(θ)=1, imply (csc θ+cot θ)(csc θ-cot θ)=1. These are the basic Pythagorean Conjugates only because these Pythagorean Conjugates can be easily tranformed another form by the multiplication or division of suitable trigonometric function accordingly, Imply 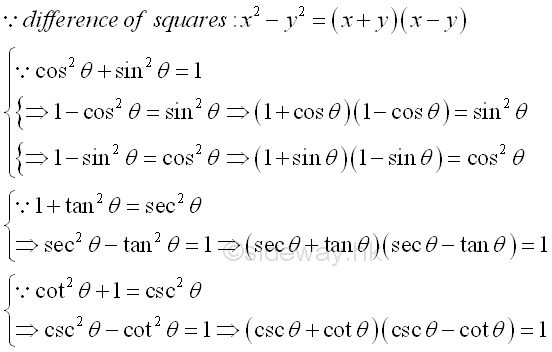
The Angles Sum and Difference Identities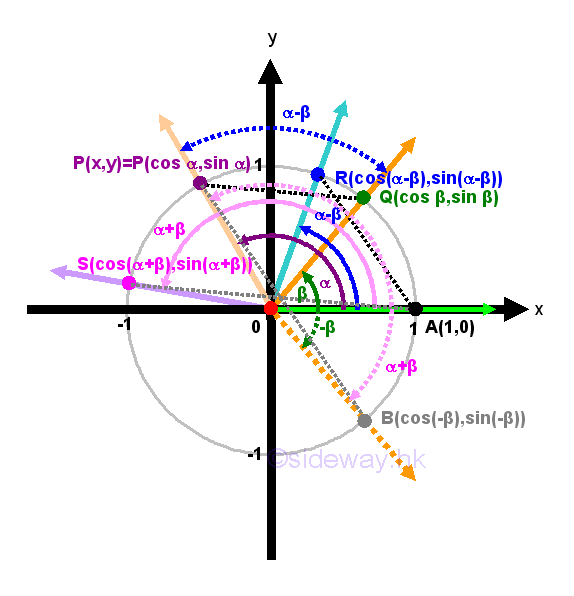
The angles
sum and difference identities can be represented using the unit circle
definition of trigonometric functions. Consider the angle difference identities
in the Cartesian coordinate system. Let P, Q, R, and A be intersection points
on unit circle where the coordinates of points are represented by the angles
included by the terminal sides of the cooresponding angle. Let ∠POA be α and ∠QOA be β
with α>β, then ∠POQ be equal α-β. Let ∠ROA be α-β. By SAS condition, ΔPOQ and ΔROA
are congruent triangles, the lengths or distances of PQ and RA are equal.
Equating the two lengths by distance formula or from Pythagorean theorem, then
√((cos β-cos α)2+(sin β-sin α)2)=√((1-cos(α-β))2+(0-sin(α-β))2) 
The angles sum and difference identities for sine function can also be obtained by the another sets of angles sum and difference with respect to the positive y-axis instead of positive x-axis such that the distance between points are difference along with the cofunction identities. Imply 
The paired distances to be considered are distances between points C and R and points P and Q', and distances between points C and S and points P and B'. The angles sum and difference identities for sine function can then be obtained through equating the two distance formulas similarly. Imply 
Indeed, the angles sum and difference identities for sine function can be derived from the angles sum and difference identities for cosine function using the cofunction identities. Imply 
Similarly, the angles sum and difference identities for tangent function can also be derived from the angles sum and difference identities for sine and cosine functions using the cofunction identities. Imply 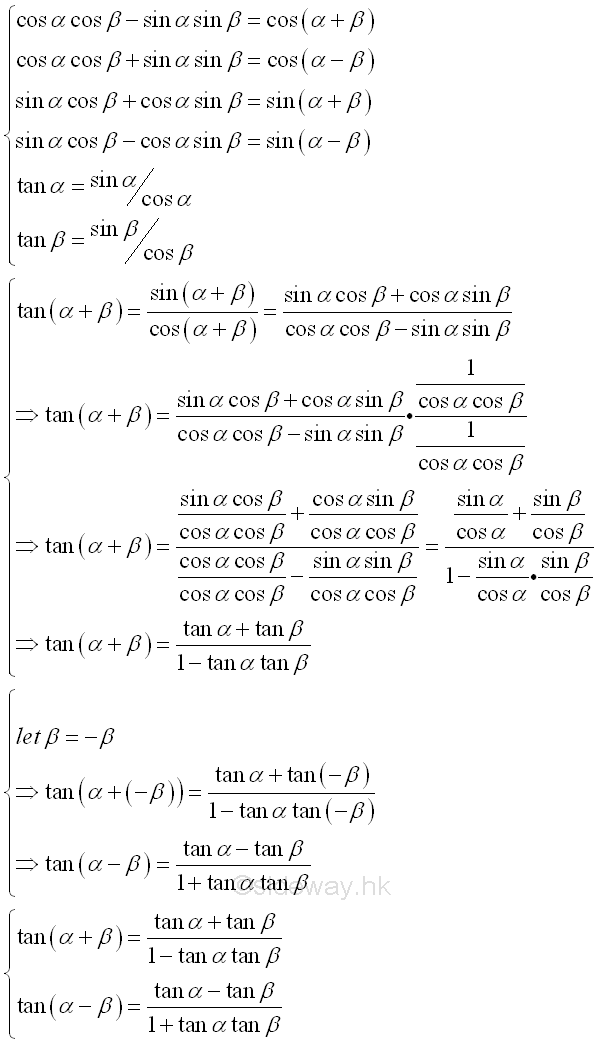
|
Sideway BICK Blog 05/06 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

