 Sideway
BICK BLOG from Sideway
Sideway
BICK BLOG from Sideway
|
Link:http://output.to/sideway/default.asp?qno=130600034 Radius of Incircle, Radius of Excircle, Laws and Formulas, Properties of Trigonometric Functions TrigonometryTrigonometric functions are related with the properties of triangles. Some laws and formulas are also derived to tackle the problems related to triangles, not just right-angled triangles. Radius of Incircle
Consider a circle incscrbed in a triangle ΔABC with centre O and radius r, the tangent function of one half of an angle of a triangle is equal to the ratio of the radius r over the sum of two sides adjacent to the angle. Since the incircle for the three different types of angled triangles can be constructed in similar way, the proofs for acute angled triangle with all the internal angles are less than π/2, the right angled triangle with one of the internal angles is equal to π/2, and the obtuse angled triangle with one of the internal angles is greater than π/2 but less than π are the same. For a circle incscrbed in a triangle ΔABC with centre O and radius r, three triangles, ΔAOB, ΔBOC, and ΔCOB, can be constructed by joining the vertices, A, B, and C to the intersection of the three angle bisectors of the triangle, O with the same altitude of r. Imply 
Consider triangle ΔCOB, imply 
Radius of Excircle
Unlike incirlce of a triangle, an excircle is constructued outside the triangle with one side and two extended lines of the triangle are tangent to the circle. The tangent function of one half of an angle of a triangle is equal to the ratio of the radius r of the circle tangent to the side opposite over the sum of three sides. Since the excircle for the three different types of angled triangles can be constructed in similar way, the proofs for acute angled triangle with all the internal angles are less than π/2, the right angled triangle with one of the internal angles is equal to π/2, and the obtuse angled triangle with one of the internal angles is greater than π/2 but less than π are the same. But the excircle tangent to each side of the triangle may not be of the same radius, the three excircles are therefore of centres Oa, Ob, and Oc and with radius r1, r2, and r3. Besides each excircle can be expressed in terms of the three angles of the triangle separately. Imply 
Consider excircle tangent side b, imply 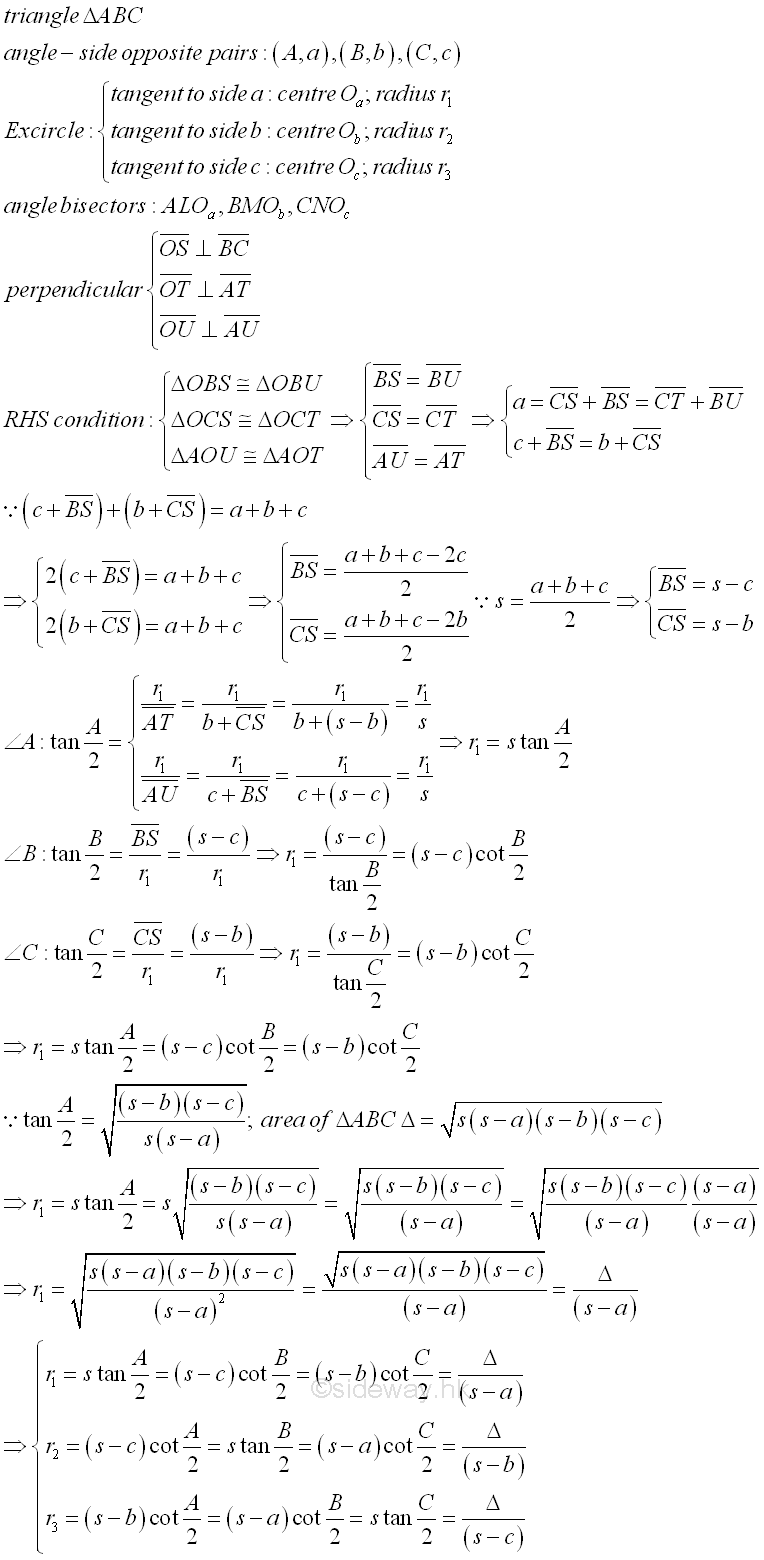
Link:http://output.to/sideway/default.asp?qno=130600035 HTML Alphabet Numbering in Unicode html ENTITIES Alphabet Numbering in Unicode
|
Sideway BICK Blog 27/06 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

