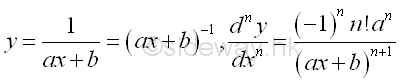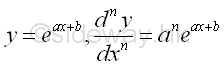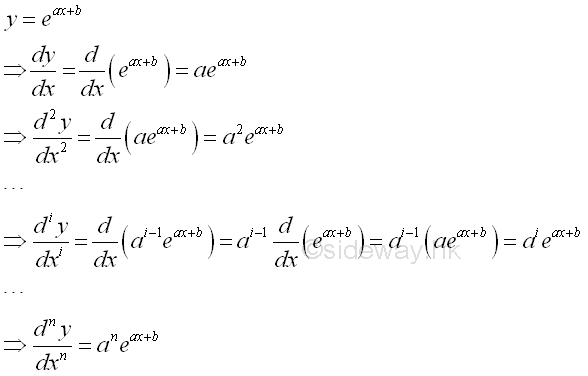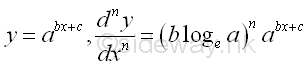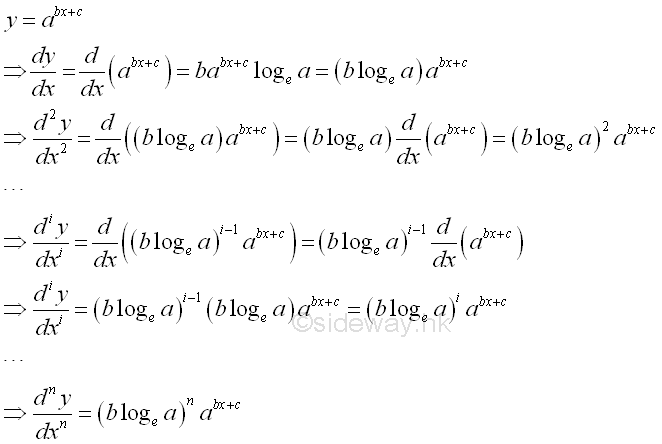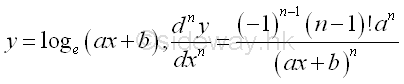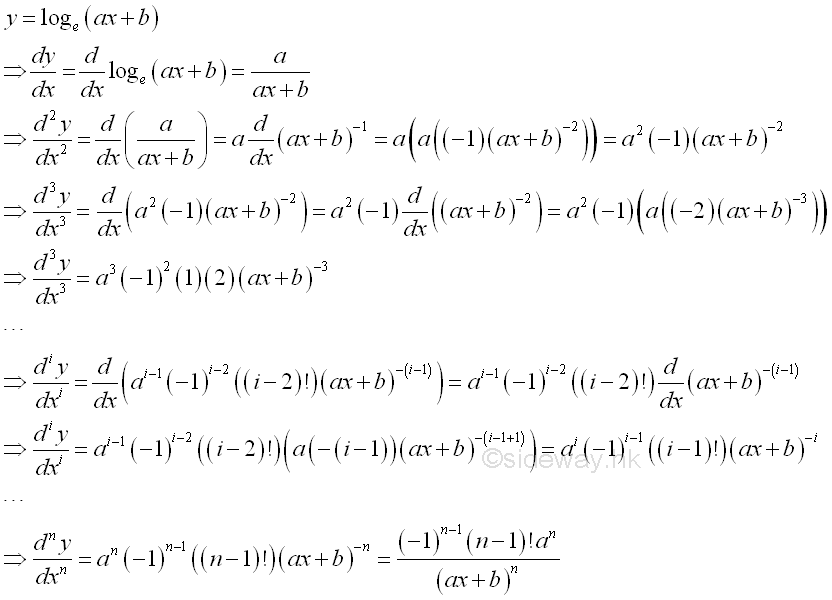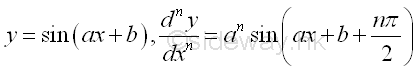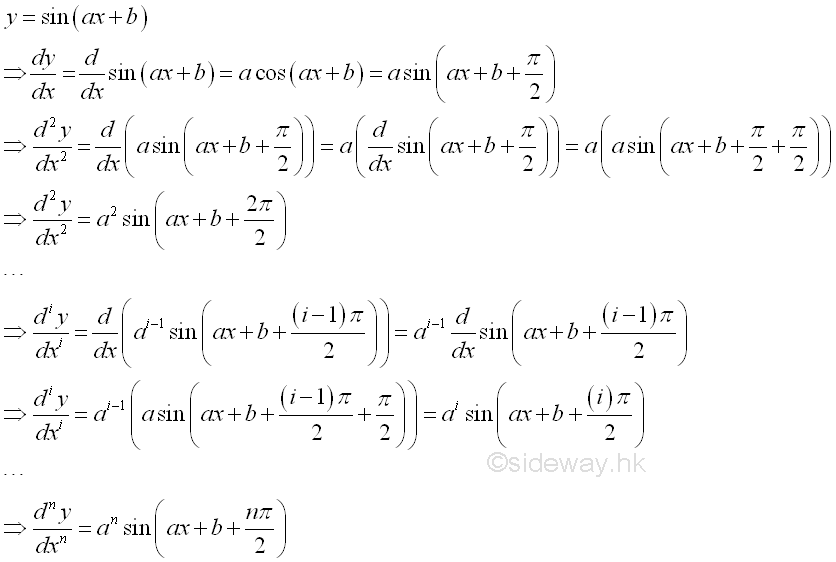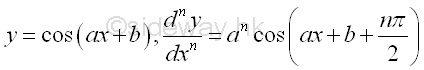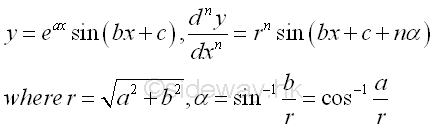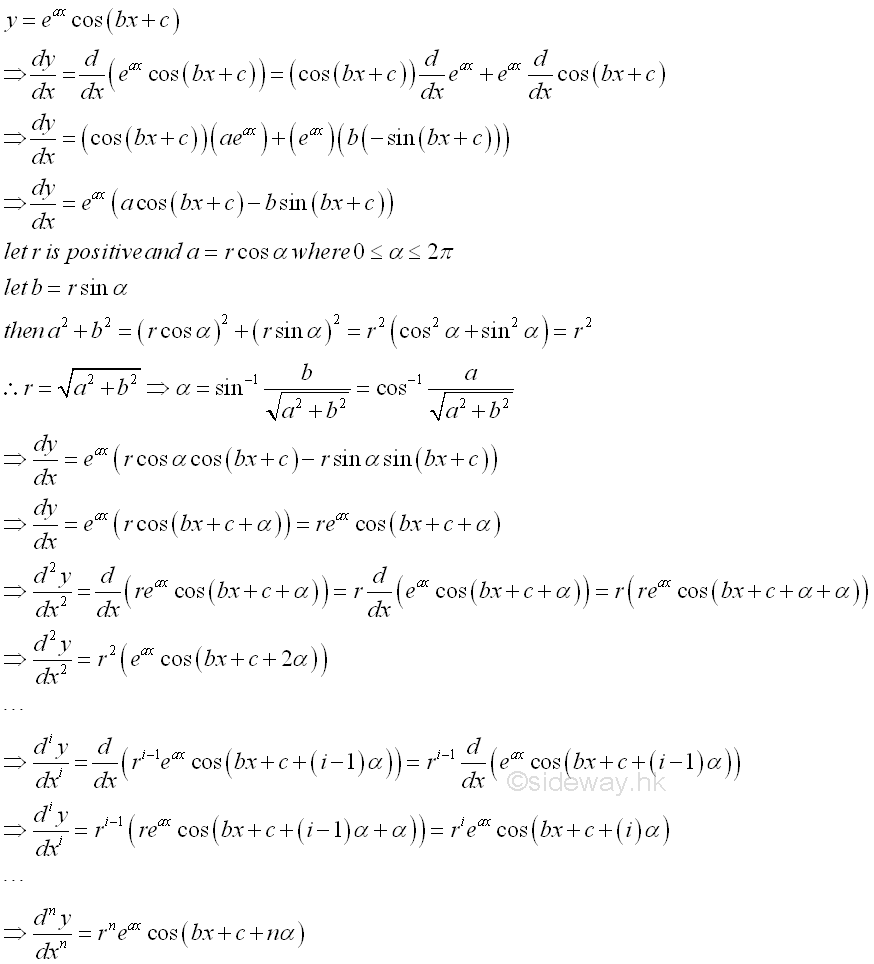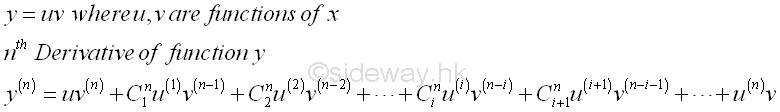Successive Differentiation

In general, the differentiation of a function can be differentiated succesively to obtain Higher Order Derivatives. Let function y=f(x) be a function of x. Then the result of differentiating y with respect to x is defined as the derivative or the first derivative of y with respect to x and is denoted as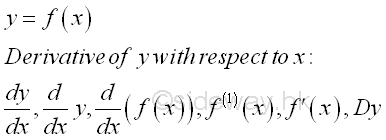

Then the result of differentiating ƒ′(x) with respect to x is defined the second differential derivative of y with respect to x and is denoted as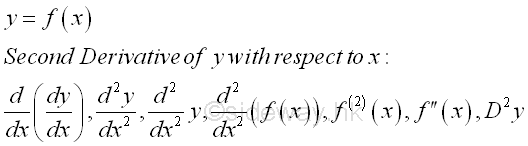

Similarly, the result of differentiating ƒ″(x) with respect to x is defined the third differential derivative of y with respect to x and is denoted as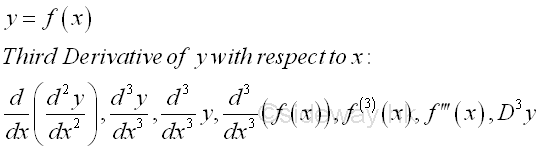 

By continuing the differentiation process, the result of differentiating ƒ(x) n times with respect to x successively is defined the nth differential derivative of y with respect to x and is denoted as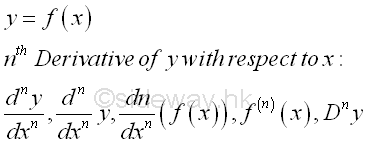 

The second, third, fourth, ..., nth derivatives are called higher order derivatives. 

nᵗʰ Derivative of Function

1. nth Derivative of General Polynomial Function

   

   Proof:

   

2. nth Derivative of General Natural Exponential Function

   

   Proof:

   

3. nth Derivative of General Exponential Function

   

   Proof:

   

4. nth Derivative of General Natural Logarithmic Function

   

   Proof:

   

5. nth Derivative of General Sine Function

   

   Proof:

   

6. nth Derivative of General Cosine Function

   

   Proof:

   

7. nth Derivative of Product of General Functions

   

   Proof:

   

8. nth Derivative of Product of General Functions

   

   Proof:

   

9. nth Derivative of Product of Functions (Leibnitz's Theorem)

   

   Proof:

   

List of Books, U

The list of Books, U in alphabetic order: