 Sideway
BICK BLOG from Sideway
Sideway
BICK BLOG from Sideway
|
Link:http://output.to/sideway/default.asp?qno=120100013 Anti-derivative of Physical Quantity Anti-derivative of Physical QuantityIn practical application, the interpretation of an indefinite integral as the anti-derivative of a function provides a mean for determining the physical qunatity when the physical quantity can be quantified in the form of infinitesimal elements. 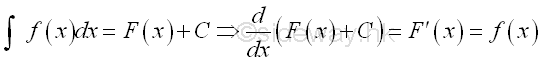
Anti-differentiation can also be applied to higher derivative. Through successive integration, related physical quantity functions can be determined. 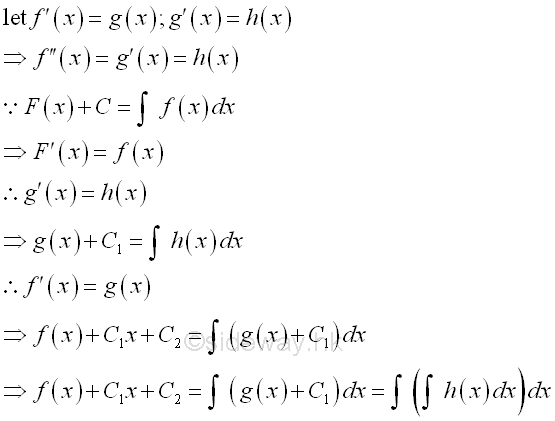
In order to determine the integration of constants, extra information, such as initial conditions, or boundary conditions are needed. Linear MotionThe four variables to describe linear motion are acceleration a, velocity v, displacement s, and time t. Imply 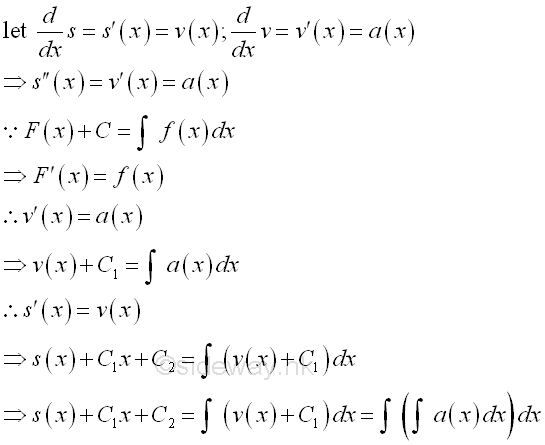
From the definition of acceleration, uniform acceleration is defined as the average velocity of an object over a period of time. imply 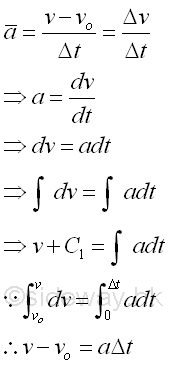
From the definition of velocity, uniform velocity is defined as the average displacement of an object over a period of time. imply 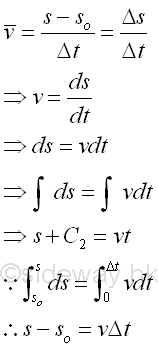
But for velocity with acceleration or deceleration, variable velocity is also a function of time. imply 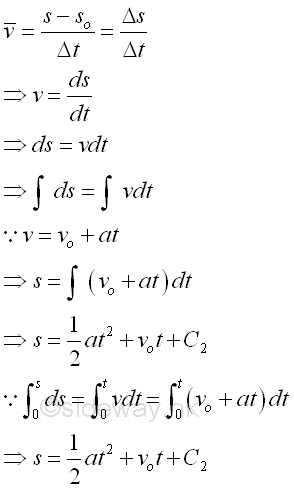
Example of Linear motionA bullet is fired to a fixed board of thickness 5. The entering speed, vo is 10 and the leaving speed, v is 2. If the deceleration, a of the bullet is a constant, how much time, t is required to pass through the board and what it the thickness of the board required to stop the bullet from coming out. Determine the acceleration first. 
Since the velocity is decelerating, the velocity is a function of time, the time required is 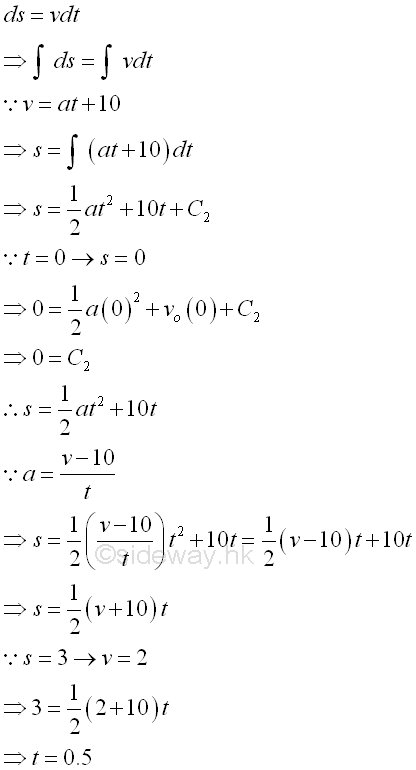
To determine the required thickness of the board to stop the bullet, determine the accelaration first, imply 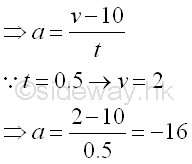
Using the same board to stop the bullet, imply v=0, and accelerarion is the same imply 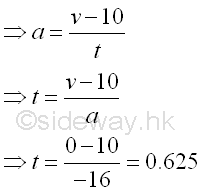
The required thickness is 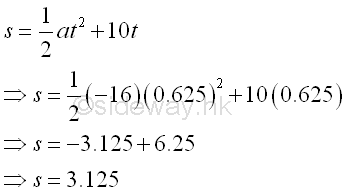
|
Sideway BICK Blog 11/01 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

