 Sideway
BICK BLOG from Sideway
Sideway
BICK BLOG from Sideway
|
Link:http://output.to/sideway/default.asp?qno=120600004 Centroid of Plane Body Centroid of 2D Plane BodyThe centroid of an plate is determined by the first moment of a two dimensional plane body with the method of the first moment of area. 
And the centroid of a wire is determined by the first moment of a two dimensional plane body with the method of the first moment of line. 
Centroids of AreasThe using of unit elemental areas of an object to determine the centroid of a 2D plane area can be expressed as 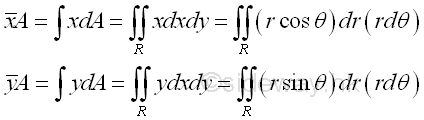
A double integation is needed to evaluate with respect to the two varables. Similar to finding the area of a 2D plane object, the centroid of an area can usually be determined by performing a single integration also. Centroid by Single IntegrationThe unit elemental areas of an object used to determine the centroid of a 2D plane area can be rearranged into grouped elemental areas. Imply 
After the grouping of unit elemental areas into one elemental area, the coordinates of the centroid of an area can also be determined by one single integration in a similar way by considering the centroid of each elemental area strip. Imply 
Centroid of Area by Single Integration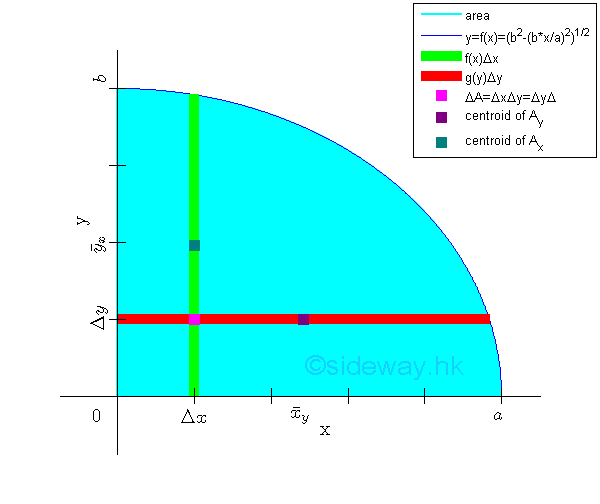
For example, the signed area of the planar region R is bounded by curves in rectangular form , Imply 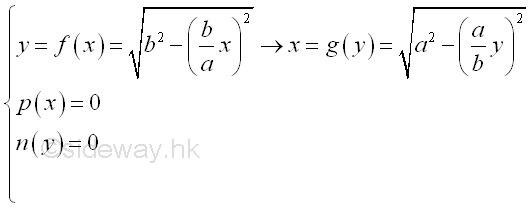
The unit element area of a region can be grouped into either a thin vertical rectangular strip or a thin horizontal rectangular strip. And the elemental area ΔA becomes 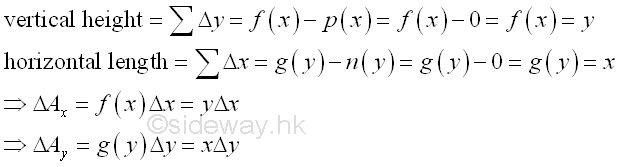
Considering the thin rectangular strip as the elemental area, the centroid of the planar region can be determined by a single integration through sweeping the elemental centroid of the elemental area strip along either rectangular coordinate axis accordingly. Imply By sweeping the centroid of horizontal strip along y axis vertically Centroid of horizontal strip. Imply 
Therefore, centroid of the bounded area is 
By sweeping the centroid of vertical strip along x axis horizontally Centroid of vertical strip. Imply 
Therefore, centroid of the bounded area is 
|
Sideway BICK Blog 04/06 |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

